AQA GCSE Gears and moments(Physics)
Gears
A gear is used to change the moment of a turning effect.


Different sized gear cogs.
The larger the diameter of the gear cog, the greater the distance between the pivot and where the line of action of the force will be.
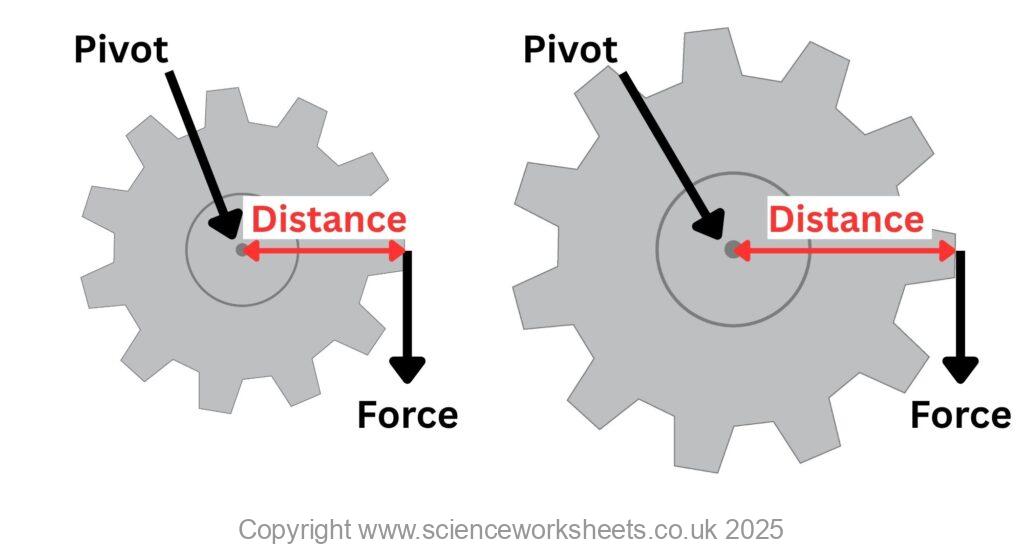
As the distance increases, then the size of the moment will increase.
So, a larger cog, will have a bigger moment.
Calculating Moments using gear cogs.
If we look at an example of a small cog turning a large cog:
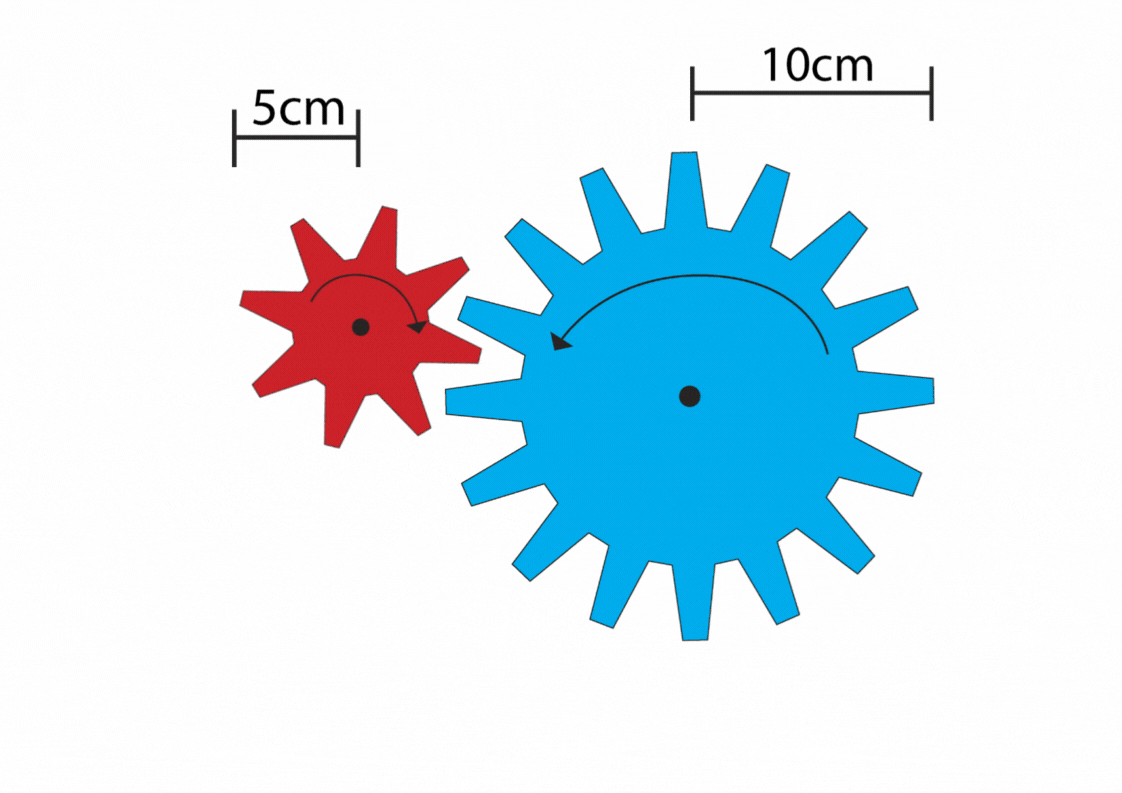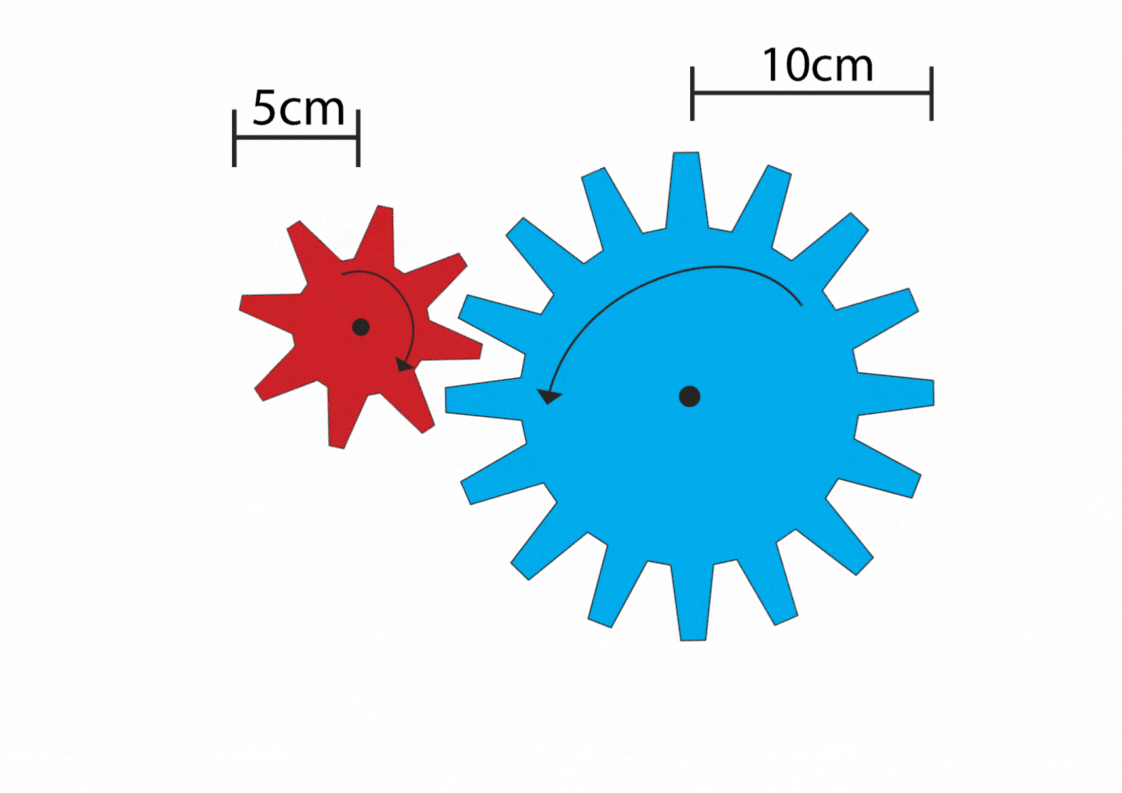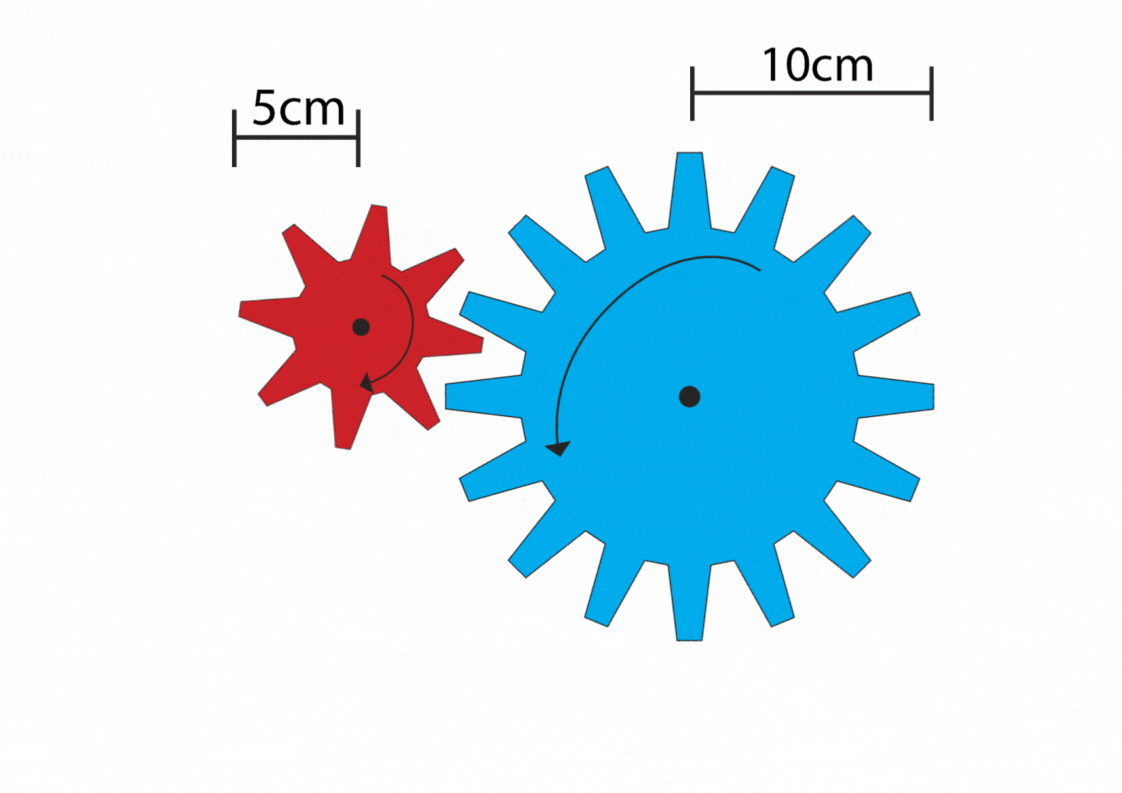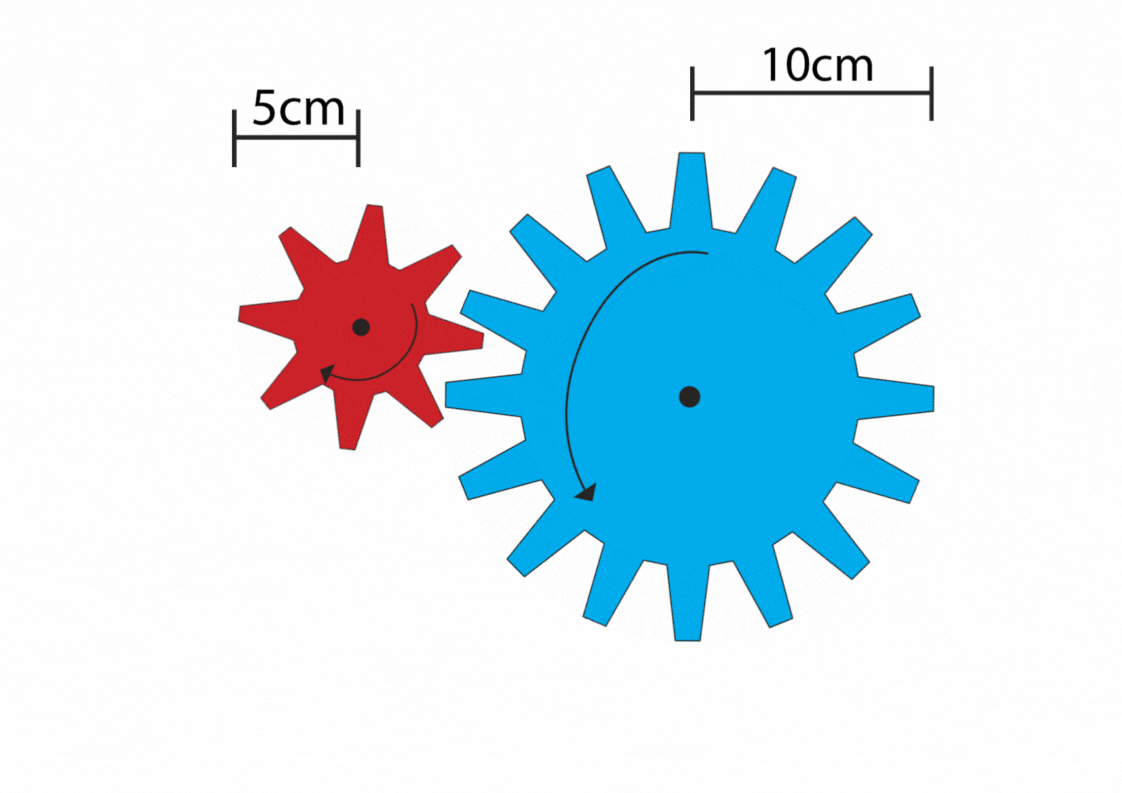
A force of 40N is applied to the small cog. Calculate the moment that the small cog produces.
5cm = 0.05m
Clockwise moment of small cog = 0.05m x 40N = 2Nm
Remember on the previous page that the forces on each gear cog are equal, but opposite in direction. This means that the large cog also experiences a 40N force.
10cm = 0.1m
Anticlockwise moment of large cog = 40N x 0.1m = 4Nm
If we look at our original defintion of a gear from the top of this page “A gear is used to change the moment of a turning effect. “
The gear cog has increased the moment in this case. So, the turning effect has increased.
If you started with a large cog, which turns a small cog, then the output moment will be smaller than the input moment.
Practice questions
1.State the definition of a gear
2.Describe the relationship between size of gear cog and the magnitude of the moment produced
3. A small gear cog is 5cm in diameter and is turned with a force of 40N. This small cog turns a larger cog which has a diamter of 20cm. Calculate the size of the output moment from the larger gear cog.
Absorption and Emission of EM Radiation
JJ Thomson and Plum pudding model
Ernest Rutherford and the Nuclear Model
Niels Bohr changing the Nuclear Model
Discovering the Proton and Neutron
Measuring radiation from radioactivity
Radiation types and properties
Random nature of radioactive decay
Radioactive contamination or irradiation
Hazards of contamination and irradiation
Studies on the effects of radiation on humans
Different half lives of radioactive isotopes
Nuclear Fission Chain Reaction
Writing nuclear fission equations
Drawing ray diagrams for a concave lens
Drawing Ray Diagram to produce a virtual image for a convex lens
Drawing ray diagram to produce a real image for a convex lens.
Specular and Diffuse Reflection
Seeing Coloured Objects Part 2
Viewing objects through coloured filters
Transparent, Translucent and Opaque