AQA GCSE Balancing Moments made more complex(Physics)
Balancing Moments made more complex
If you have not read our balancing moments page, please read this one first.
Calculations using Principle of Moments
Force 1, F1 x distance 1, d1 = Force 2, F2 x distance 2, d2
Example calculation
Use the diagram below to answer the following question
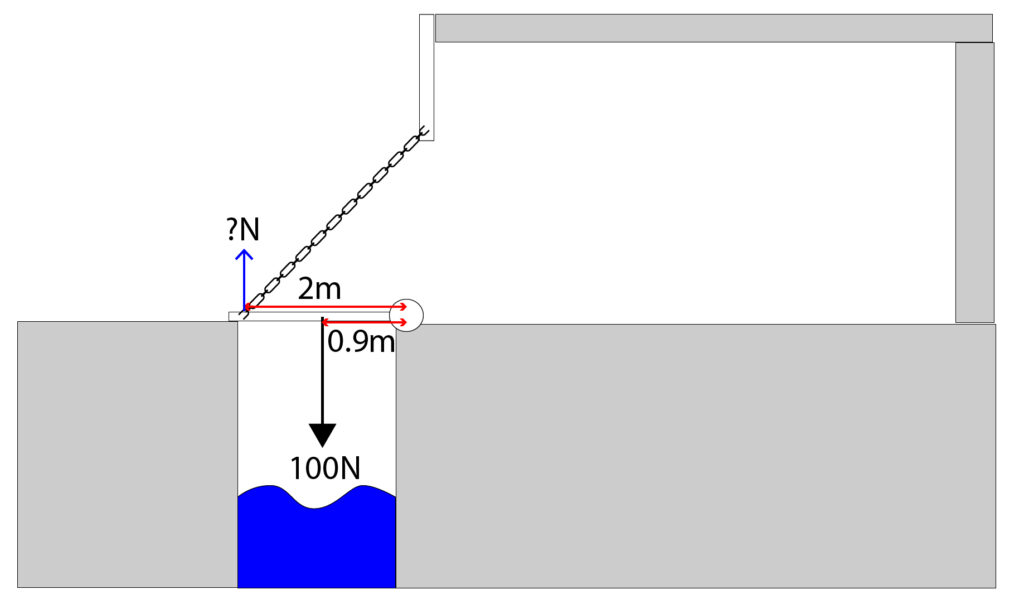
Calculate the upward force that is exerted on the drawbridge when it is lifted upwards.
The pivot this time is at the far right and is marked by a circle. Lets draw a diagram that shows both the anticlockwise and clockwise moments.
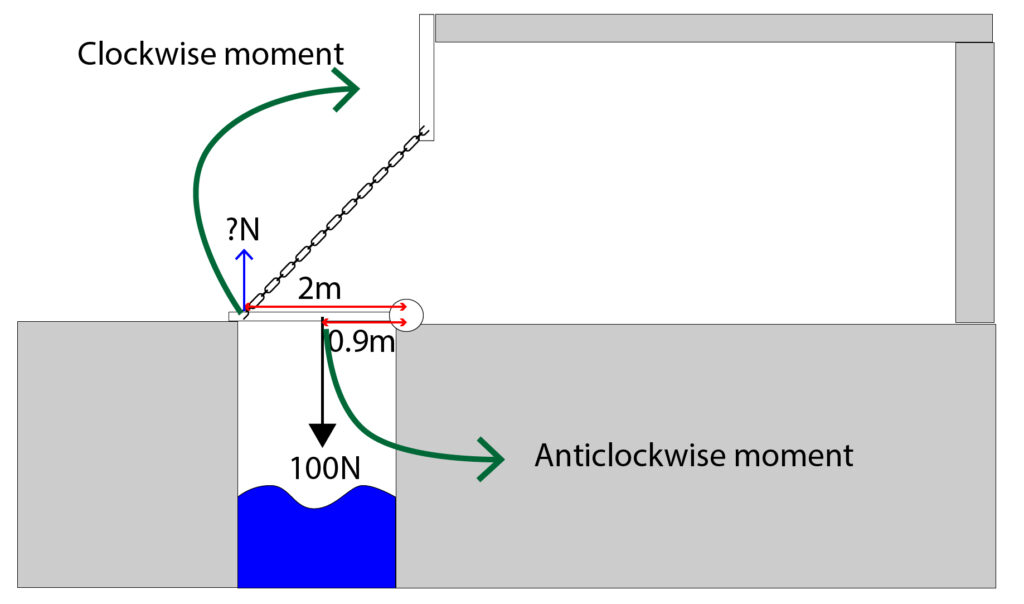
F1 = 100N, d1 = 0.9m, F2=?N, d2 = 2m
Force 1, F1 x distance 1, d1 = Force 2, F2 x distance 2, d2
100N x 0.9m = ?N x 2m
?N = (100N x 0.9m)/2m
?N = 45N
Example question 2
Use the image below to answer the following question
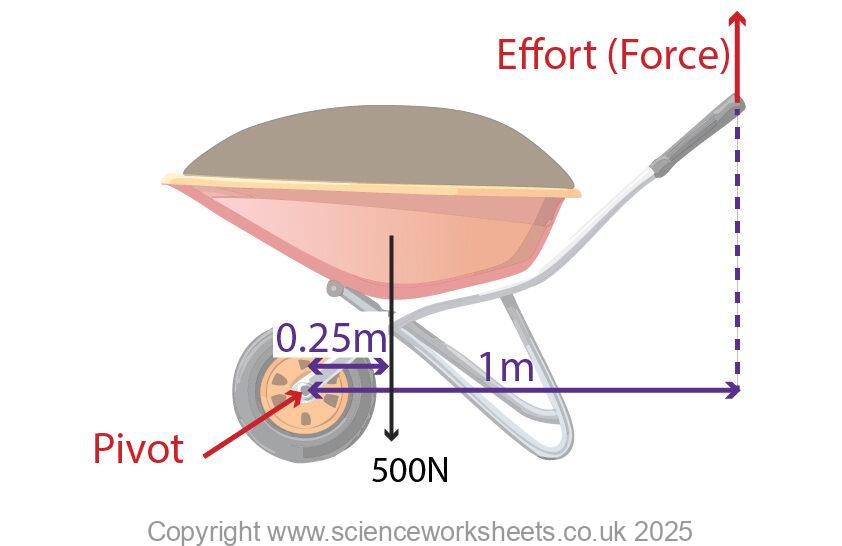
Calculate the size of the minimum upward force needed to lift the wheelbarrow.
First lets look at the position of both the clockwise and anticlockwise moments.
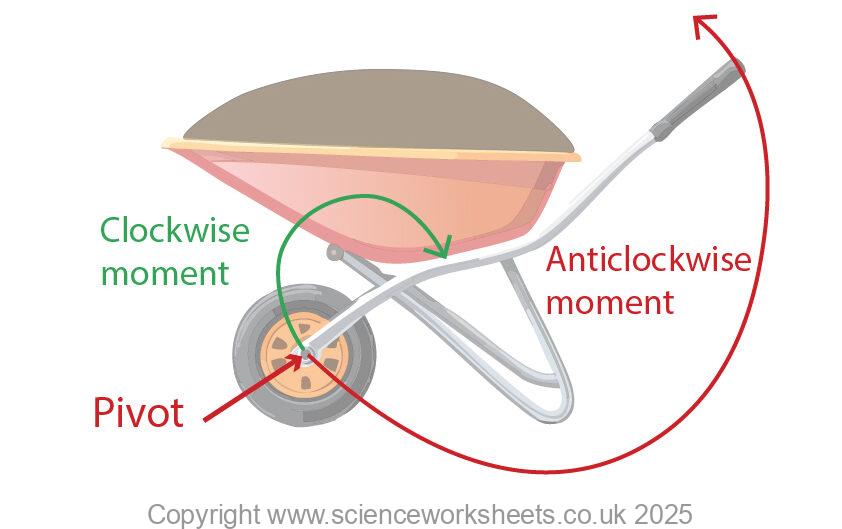
Ignore the size of the arrows, diagram above is only to ilustrate the idea of both anticlockwise and clockwise moments. The other labels were removed to make the diagram easier to visualise.
Force 1, F1 x distance 1, d1 = Force 2, F2 x distance 2, d2
F1 = 500N, d1 = 1m, F2=500N, d2 =0.25m
500N x 0.25m = ?N x 1m
?N = (500N x 0.25m)/1m
?N = 125N
Practice questions
1.Use the image below to answer the following questions

The weight of the box on the trolley is 500N. The line of action for the weight force is 0.25m from the pivot. A vertical force is applied to the handle that is 1.3m from the line of action of vertical force to the line of action of the weight force for the box to hold the trolley stationary.
Calculate the size of the force F2.
2.Use the image below to help you to answer the following question.
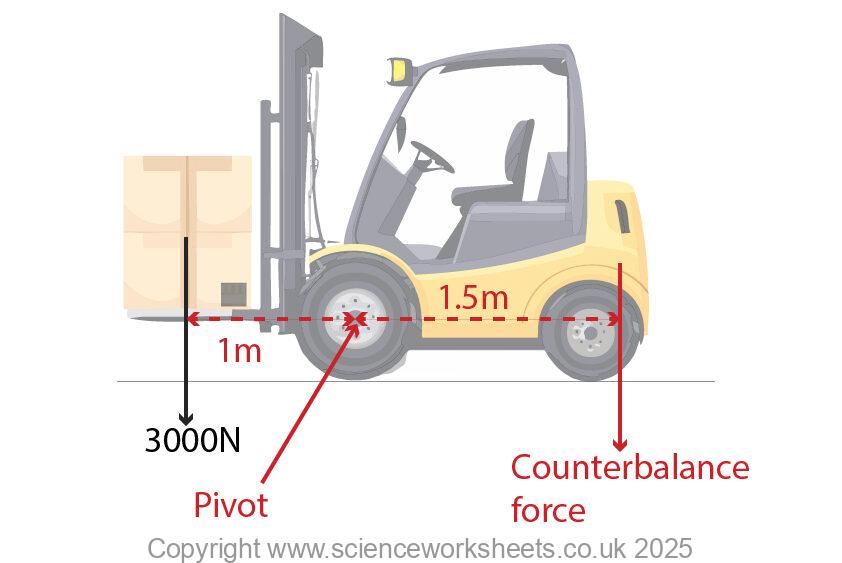
Calculate the size of the counter balance force.
3. Use the image below to answer the following question.
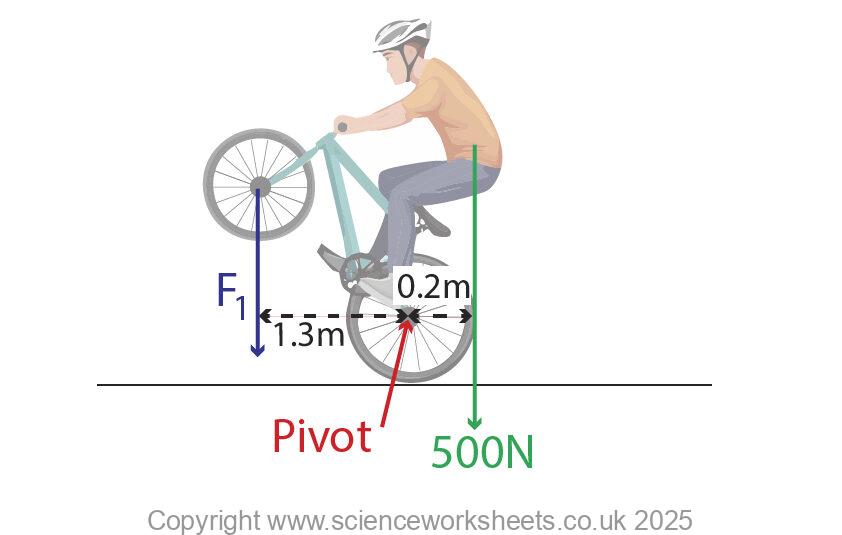
The position of the pivot is on the back wheel. There is a distance of 0.2m from pivot to the line of action for the force of 500N. There is a distance of 1.3m from the pivot to the line of action for F1.
Calculate the size of F1.
Absorption and Emission of EM Radiation
JJ Thomson and Plum pudding model
Ernest Rutherford and the Nuclear Model
Niels Bohr changing the Nuclear Model
Discovering the Proton and Neutron
Measuring radiation from radioactivity
Radiation types and properties
Random nature of radioactive decay
Radioactive contamination or irradiation
Hazards of contamination and irradiation
Studies on the effects of radiation on humans
Different half lives of radioactive isotopes
Nuclear Fission Chain Reaction
Writing nuclear fission equations
Drawing ray diagrams for a concave lens
Drawing Ray Diagram to produce a virtual image for a convex lens
Drawing ray diagram to produce a real image for a convex lens.
Specular and Diffuse Reflection
Seeing Coloured Objects Part 2
Viewing objects through coloured filters
Transparent, Translucent and Opaque