Answers to AQA GCSE Half Life Calculations

Practice Questions

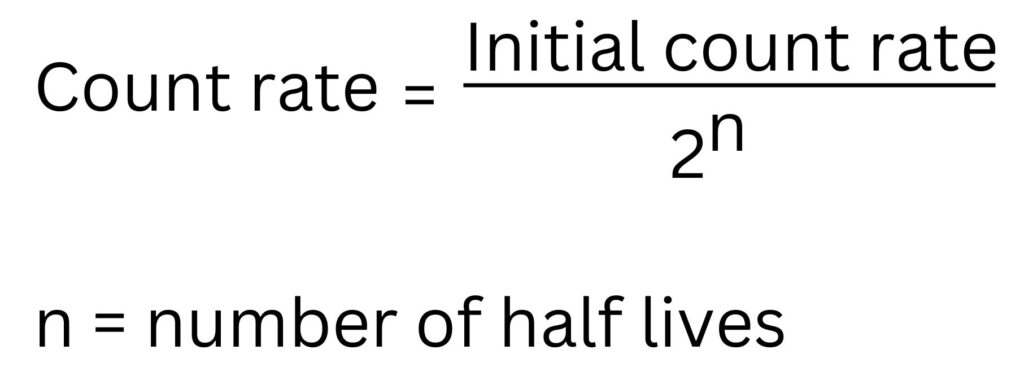
1. Polonium-210 has a half life of 140 days. If an original sample of Polonium-210 has an activity of 500Bq what will its activity be after 560 days.

560 days/140 days = 4 half lives

Count rate = Initial count rate/2ⁿ

Count rate = 500/2⁴

Count rate = 31.25

2. A radioactive isotope undergoes a series of half lives. At the start there are 8 x 10³⁶ atoms of the isotope present. At a point later in time there are 1 x 10³⁶ atoms remaining. Calculate the net decline.

Final number of radioactive atoms :Initial number of radioactive atoms

net decline = 1 x 10³⁶ : 8 x 10³⁶

net decline = 1:8

net decline = 1:8

3. If the initial count rate is 200,000Bq. Calculate the net decline after 4 half lives

The count rate will need to be halved 4 times

200,000/2 = 100,000Bq

100,000/2 = 50,000Bq

50,000/2 = 25,000Bq

25,000/2 = 12,50Bq

So, after 4 half lives the count rate is 12,500Bq

net decline = Final count rate:Initial count rate

net decline = 12500:200000

net decline = 125:200

net decline = 25:40

net decline = 5:8